-
For any point
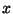 on our surface there is a open set
on our surface there is a open set 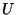 containing
containing 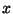 and a
homeomorphism
and a
homeomorphism 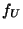 from
from 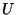 to the real projective plane
to the real projective plane 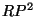 . The pair
. The pair 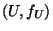 is called a chart.
is called a chart.
-
If
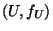 and
and 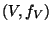 are charts with
are charts with  non-empty then we further require that
non-empty then we further require that
 acts projective linearly on
acts projective linearly on 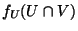 .
(That is
.
(That is
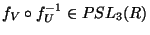 ) These functions are called transition functions.
) These functions are called transition functions.
We can similarly define a hyperbolic structure on a surface, by defining charts as maps from open sets to the hyperbolic plane ![]() and requiring that the transition functions be hyperbolic isometries
and requiring that the transition functions be hyperbolic isometries
![]() .
.
Given a real projective or hyperbolic structure on a surface ![]() ,
through analytic continuation, we can define the developing map
,
through analytic continuation, we can define the developing map ![]() ,
a map from the universal cover
,
a map from the universal cover ![]() into
into ![]() or
or ![]() respectively.
respectively.
![]() acts in the obvious way on
acts in the obvious way on ![]() and in addition we
get a representation
and in addition we
get a representation ![]() of the fundamental group
of the fundamental group ![]() into
into
![]() or
or
![]() so that
for all
so that
for all
![]() ,
,
![]() .
.
 |
| A pair of pants decomposition of a genus two surface |
 |
 |
| Constructing a pair of pants from two right hexagons |
 |
| Dehn Surgery on a surface |
Now lets return to projective geometry. We start with hyperbolic structures on 3-punctured spheres. These hyperbolic structures give us projective structures via the Klein model. Now we would like to glue 3-punctured spheres together. At this point it is not obvious what gluing freedoms we have available. For instance, can we glue two such projective 3-punctured spheres together in such a way as to get a new projective structure on a surface which is not induced by a hyperbolic structure? To answer this question, we must return to our discussion of the developing map.
Suppose we cut a surface ![]() with a hyperbolic structure along an embeded geodessic loop
with a hyperbolic structure along an embeded geodessic loop ![]() in the interior of our surface dividing the surface into two pieces,
in the interior of our surface dividing the surface into two pieces, ![]() and
and ![]() . In
. In ![]() this loop lifts to several geodessics, pick one and call it
this loop lifts to several geodessics, pick one and call it ![]() . We have the induced projective structure on this surface as well.
We know from our description of the Klein model that
. We have the induced projective structure on this surface as well.
We know from our description of the Klein model that ![]() is a line in
is a line in ![]() dividing the interior of the conic into two disjoint pieces.
dividing the interior of the conic into two disjoint pieces.
Now consider cutting
![]() along
along ![]() and then regluing.
We can take a closed collar nieghborhood of
and then regluing.
We can take a closed collar nieghborhood of ![]() and look at its boundary components, call them
and look at its boundary components, call them ![]() and
and ![]() and assume that they are homotopically equivalent (in
and assume that they are homotopically equivalent (in ![]() ,
,
![]() ) and lie in
) and lie in ![]() and
and ![]() resepectively. Choose the right lifts
resepectively. Choose the right lifts
![]() and
and ![]() to
to ![]() and they will lie in seperate pieces of
and they will lie in seperate pieces of
![]() \
\![]() . Now instead of regluing exactly the way the surface was before consider deforming the second piece by a transformation
. Now instead of regluing exactly the way the surface was before consider deforming the second piece by a transformation
![]() then regluing, that is a point
then regluing, that is a point ![]() in this piece are first sent to
in this piece are first sent to ![]() and then we reglue the image. We obviously would like
and then we reglue the image. We obviously would like ![]() to preserve the line segment but we require a little more.
to preserve the line segment but we require a little more.
Because ![]() and
and ![]() are homotopically equivalent,
in terms of the representation
are homotopically equivalent,
in terms of the representation ![]() ,
,
![]() .
So to glue in the way we described above, we need that
.
So to glue in the way we described above, we need that
![]() .
One way to ensure that this holds, is to ensure that
.
One way to ensure that this holds, is to ensure that ![]() commutes with
commutes with
![]() .
.
Notice that if
![]() is an hyperbolic action on the Klein model, it will fix a line inside
the conic containing the hyperbolic plane. In particular, it will also
fix the two points
is an hyperbolic action on the Klein model, it will fix a line inside
the conic containing the hyperbolic plane. In particular, it will also
fix the two points ![]() and
and ![]() at which this line intersects the conic boundary. Also because it fixes the conic, it fixes the two lines
at which this line intersects the conic boundary. Also because it fixes the conic, it fixes the two lines ![]() and
and ![]() tangent to the conic at these point, and therefore also fixes the point at which they meet,
tangent to the conic at these point, and therefore also fixes the point at which they meet, ![]() . Recall that two projective transformations commute if and only if they have the same three fixed points.
. Recall that two projective transformations commute if and only if they have the same three fixed points.
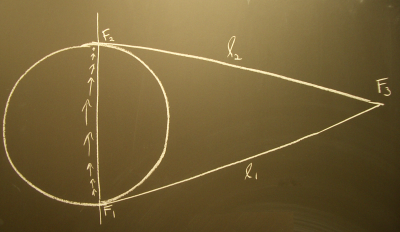 |
| Fixed points of a hyperbolic translation in the Klein Model |
So we only consider use of ![]() which fix the three fixed points described above. The space of projective transformations which fix these is a
which fix the three fixed points described above. The space of projective transformations which fix these is a ![]() -dimensional set. After choosing such a
-dimensional set. After choosing such a ![]() and performing our gluing operation, we must also must reglue the rest of the images under the developing map of preimages of
and performing our gluing operation, we must also must reglue the rest of the images under the developing map of preimages of ![]() in
in ![]() in a similar way. After regluing in this way, there will be a new convex set invariant under
in a similar way. After regluing in this way, there will be a new convex set invariant under
![]() often not the interior of a conic.
often not the interior of a conic.
The images of our conic under maps ![]() can be any conic tangent to
can be any conic tangent to ![]() and
and
![]() at the points
at the points ![]() and
and ![]() respectively. Our two dimensional space of deformations we can make in
this manner, can be thought of as decomposing into two directions, one
with hyperbolic deformations (Dehn surgeries along
respectively. Our two dimensional space of deformations we can make in
this manner, can be thought of as decomposing into two directions, one
with hyperbolic deformations (Dehn surgeries along ![]() ) and the other projective deformations which change the shape of
) and the other projective deformations which change the shape of
![]() .
It is not necessary that the we preform the surgeries along geodessic
loops which divide the surface into two hyperbolic pieces; we can do
these surgeries whenever a surface can locally split into two
hyperbolic pieces (say into two pairs of pants).
.
It is not necessary that the we preform the surgeries along geodessic
loops which divide the surface into two hyperbolic pieces; we can do
these surgeries whenever a surface can locally split into two
hyperbolic pieces (say into two pairs of pants).
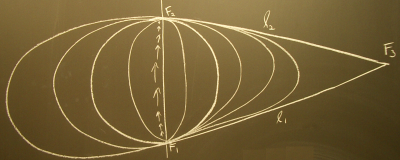 |
| Possible images of the conic under the map |
- Thurston, William P. Three-dimensional geometry and topology. Princeton University Press, Princeton, 1997.
Hopefully, more to come
The applet below allows a user to explore this space of projective deformations of a hyperbolic structure on a genus two surface by viewing the developing map. Note that the boundary of the developing map should always be a convex shape whose boundary curve is differentiable but not twice differentiable unless that boundary is a conic.
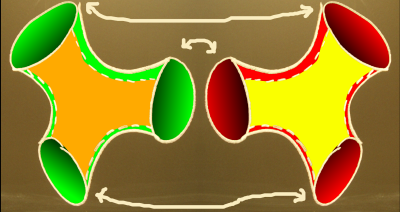 |
| The gluing of a hyperbolic surface used. Deformations happen where the two pairs of pants meet. |
The applet begins
with a hyperbolic tiling by equilateral right hexagons. Any four
adjacent differently colored right hexagons form a fundamental domain
for the group action of
![]() on the interior of the conic.
on the interior of the conic.
You can zoom in and out in the upper left hand box by holding down the mouse button and dragging up or down on the mouse in the box.
You may move the vertices of the white quadrilateral around the box on the left. Possible placements for these vertices represent maps from the interior of the quarilateral to the box on the upper right side of the screen; vertices being mapped to the corners. It is recomended that you attempt to keep the quadrilateral convex and ensure that it contains the tiling.
In the lower left, you may apply the deformations along curves decomposing the surface into a pair of pants- six deformations in all.
An important button in the lower right allows you to load the image on the right into the image on the left. This allows you to explore the infinite nature of the tilings. By choosing a view then loading the image and repeating, you can close in on any hexagon in the tiling.
The animations displayed when deforming do not represent actual projective structures on the surfaces- it is just there as a flashy effect, and is just linearly interpolating between the two images. Drawings after the animation completes however do represent projective structures on the genus two surface.
Enjoy!
Get the Source Code